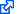A Quora question asked why, if time is just another dimension, it is not possible to travel backwards in time.

I offered three illustrations by way of an answer.

First, let me draw spacetime with one spatial dimension suppressed. In this drawing, you (the observer) sit at the origin, and the two cones represent your past and future light cone (i.e., light rays that are reaching your eye "just now", vs. light rays that you emit with a light source "just now".)

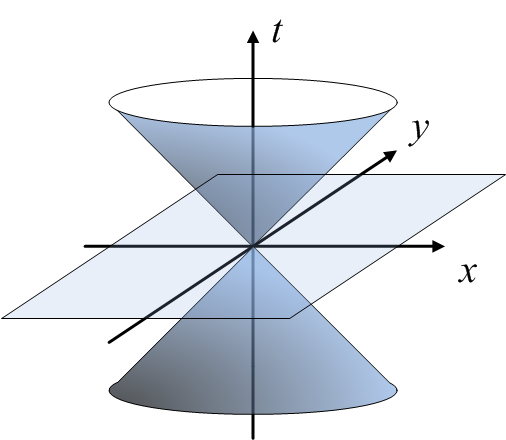
Now let me rotate my drawing in space. The time axis remains in place, as I am rotating in the xy-plane:

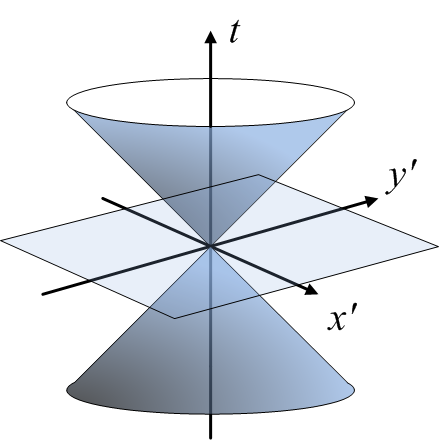
As you can see, the rotation is unconstrained. If I did a 180 degree rotation, I would have ended up with both the x and the y axis reversed.

But what happens when I rotate in such a way that it involves the time axis? Here is an example:

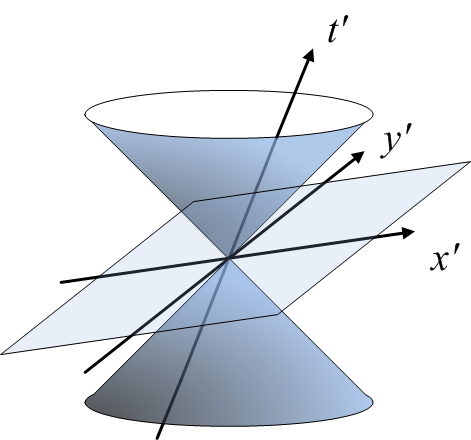
As you can see, the rotation (a hyperbolic rotation, which corresponds to a change of velocity) works in such a way that the light cones remain exactly where they were. They are invariant. (This is the geometric representation of the fact that light cones and, more generally, the laws of electromagnetism, are invariant under Lorentz transformations.)

And most importantly, what is inside the upper (or lower) light cone stays inside the upper (or lower) light cone. What is outside stays outside. And as a corollary, no matter how I perform this hyperbolic rotation or how many different ways I do it, I can never change the direction of the time axis. It will never turn upside down because for that to happen, it would have to get outside the upper light cone and then inside the lower lightcone somehow, and that is just not possible.

The time axis, of course, represents the world line of the observer at rest. (The spatial coordinates of that observer would remain unchanged, only time would pass.) So the fact that we cannot turn the time axis around is equivalent to saying that an observer can never move backwards in time. This is how the relativistic geometry of spacetime works.