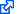Using high-school algebra, you can do all sorts of despicable things with the cubic equation $x^3+px^2+qx+r=0$.
Except solve it. No matter how you manipulate that equation, there's no way you can arrive at a solution all by yourself; not unless you happen upon the idea that by substituting $y=x+p/3$, you can in fact eliminate the quadratic component of the sum, thereby reducing the equation to the form $y^3+uy+v=0$, which is more easily solvable.
It was Cardano$^\star$ who first had this spark and solved the cubic equation.
The second trick in Cardano's solution comes after the initial substitution that eliminates the second-power component from the equation:
\begin{align}x^3+px^2+qx+r&=0,\\
y&=x+\frac{p}{3},\\
y^3+\left(q-\frac{p^2}{3}\right)y+\left(r-\frac{pq}{3}+\frac{2p^3}{27}\right)&=0.\end{align}
Substituting
\begin{align}s&=q-\frac{p^2}{3},\\t&=r-\frac{pq}{3}+\frac{2p^3}{27},\end{align}
we can write down a cubic equation for $y$ with no quadratic term:
\[y^3+sy+t=0.\]
What one must notice is that a formally identical equation can be derived by expanding the third power of a sum:
\begin{align}(u+v)^3=u^3+3u^2v+3uv^2+v^3&=u^3+3uv(u+v)+v^3,\\
(u+v)^3-3uv(u+v)-(u^3+v^3)&=0.\end{align}
From this, a system of equations for $u^3$ and $v^3$ can be derived:
\begin{align}27u^3v^3&=-s^3,\\u^3+v^3&=-t.\end{align}
This can be reduced to a quadratic equation for either $u^3$ or $v^3$. What makes this solution peculiar is that whenever the original cubic equation has three real roots, this quadratic equation has no real roots at all. In fact, it was this issue that motivated the development of the theory of complex numbers, which led, much later, to the algebra of quaternions, which provide the mathematical foundation for Dirac spinors and a lot of modern quantum physics...
Here's a good example illustrating Cardano's problem:
\[x^3-6x+4=0.\]
This equation has three real roots: $2$, $-1+\sqrt{3}$, $-1-\sqrt{3}$.
Following Cardano's method, we'd have a system of equations for $U=u^3$ and $V=v^3$ as follows:
\begin{align}27UV=216\Rightarrow UV&=8,\\U+V&=-4.\end{align}
From this, a quadratic equation for $U$ can be derived:
\begin{align}U(-4-U)&=8,\\U^2+4U+8&=0.\end{align}
Unfortunately, this equation has no real roots. For Cardano and his contemporaries, this was a huge problem. Today, we can use complex numbers to find the roots:
\[U=-2\pm\sqrt{2^2-8}=-2\pm 2i.\]
Similarly,
\[V=-2\mp 2i.\]
Taking the cubic root we get:
\begin{align}&u_1=1+i,~~~~v_1=1-i,\\&x_1=u_1+v_1=1+i+1-i=2.\end{align}
But of course in the complex plane, a number has three cubic roots, two of which can be obtained from the third by multiplying by $-\frac{1}{2}\pm\frac{\sqrt{3}}{2}i$:
\begin{align}&u_2=(1+i)\left(-\frac{1}{2}+\frac{\sqrt{3}}{2}\right),~~~~v_2=(1-i)\left(-\frac{1}{2}-\frac{\sqrt{3}}{2}i\right),\\
&x_2=u_2+v_2=-1-\sqrt{3},\\
&u_3=(1+i)\left(-\frac{1}{2}-\frac{\sqrt{3}}{2}i\right),~~~~v_3=(1-i)\left(-\frac{1}{2}+\frac{\sqrt{3}}{2}i\right),\\
&x_3=u_3+v_3=-1+\sqrt{3}.\end{align}
The bottom line: we've recovered the three real roots of the original cubic equation, but in the process, we were forced to make use of the square root of negative numbers. This was enough to give old Cardano a fit: "thus far does arithmetical subtlety go, of which this, the extreme, is, as I have said, so subtle that it is useless".
$^\star$It was Niccolò Fontana Tartaglia who solved equations in the form $y^3+sy+t=0$, the solution of which he communicated to Gerolamo Cardano. Cardano was able to reduce other cubics to this form, and his student Lodovico Ferrari used Tartaglia's method to solve the quartic equation. Cardano published all these results in his Ars Magna. Tartaglia's solution may also have been independently discovered by Scipione del Ferro.